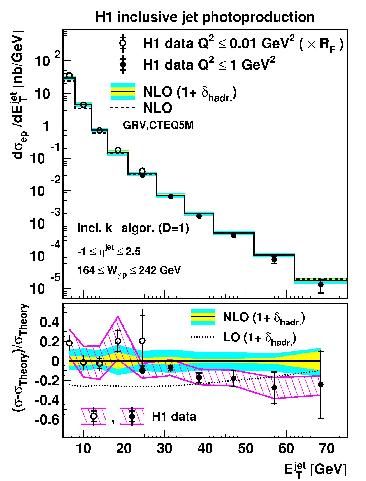
 The cross sections for jet production are measured as a function of the jet
pseudorapidity (essentially its angle in the detector relative to the beam
axis) and its energy
projection transverse to the beam axis. In order to test the theory
as closely as possible, they are also measured multi-differentially in these
variables and the photon-proton centre-of-mass energy. An example measurement
of the transverse energy dependence is shown in the figure. Very high
transverse energies of nearly 70 GeV are reached and the cross section drops
by 6 orders of magnitude over the range of measurement. As can be seen from
the bottom part of the figure, the state-of-the-art theory (`NLO' QCD) does
a very good job of reproducing this variation using appropriate sets of
quark and gluon densities for the proton and the photon. This is not the
case when the slightly less refined version of the theory (`LO' QCD) is used.
This good description of the data by NLO QCD is observed for all distributions
studied, with the possible exception of the pseudorapidity distribution at
the lowest transverse energy. Comparing the size of the errors on the data
points with the bands representing the errors on the calculations, it is clear
that theoretical improvements as well as better data are required in order to
test the theory in even finer detail.
The cross sections for jet production are measured as a function of the jet
pseudorapidity (essentially its angle in the detector relative to the beam
axis) and its energy
projection transverse to the beam axis. In order to test the theory
as closely as possible, they are also measured multi-differentially in these
variables and the photon-proton centre-of-mass energy. An example measurement
of the transverse energy dependence is shown in the figure. Very high
transverse energies of nearly 70 GeV are reached and the cross section drops
by 6 orders of magnitude over the range of measurement. As can be seen from
the bottom part of the figure, the state-of-the-art theory (`NLO' QCD) does
a very good job of reproducing this variation using appropriate sets of
quark and gluon densities for the proton and the photon. This is not the
case when the slightly less refined version of the theory (`LO' QCD) is used.
This good description of the data by NLO QCD is observed for all distributions
studied, with the possible exception of the pseudorapidity distribution at
the lowest transverse energy. Comparing the size of the errors on the data
points with the bands representing the errors on the calculations, it is clear
that theoretical improvements as well as better data are required in order to
test the theory in even finer detail.
The data in the paper are also compared with similar measurments from proton-antiproton collisions in order to study the effects arising from the different structure of the photon and proton. This is done as a function of a variable x_T, which is related to the fractions of the photon and proton momenta carried by the interacting quarks and gluons. At low values of x_T, the results from photon-proton and from antiproton-proton collisions show a remarkably similar shape, illustrating that the photon really does develop into something similar to a hadron in its quantum fluctuations. At larger x_T, where the photon acts in a more point-like way, the distributions become very different.